4
$\begingroup$

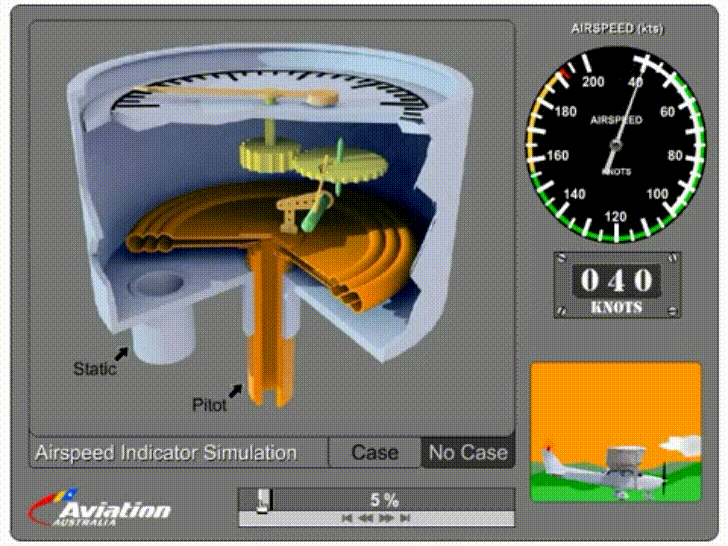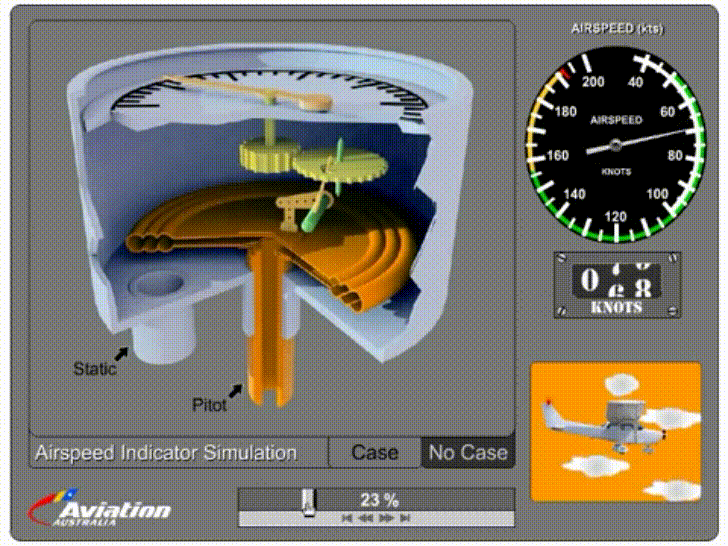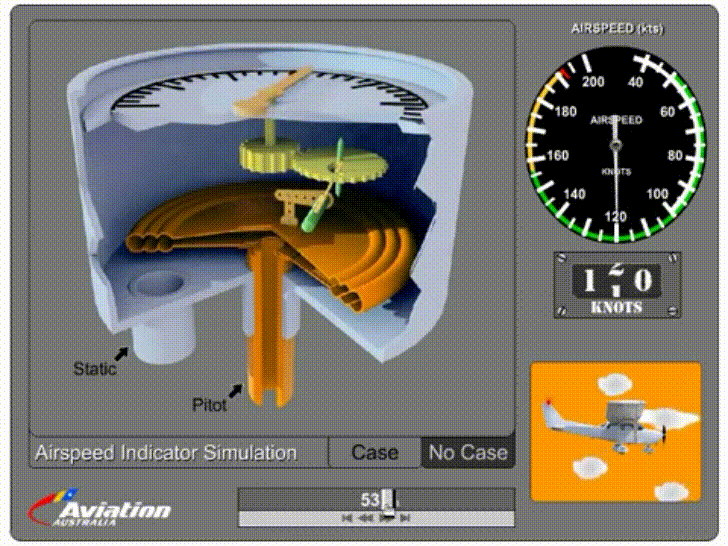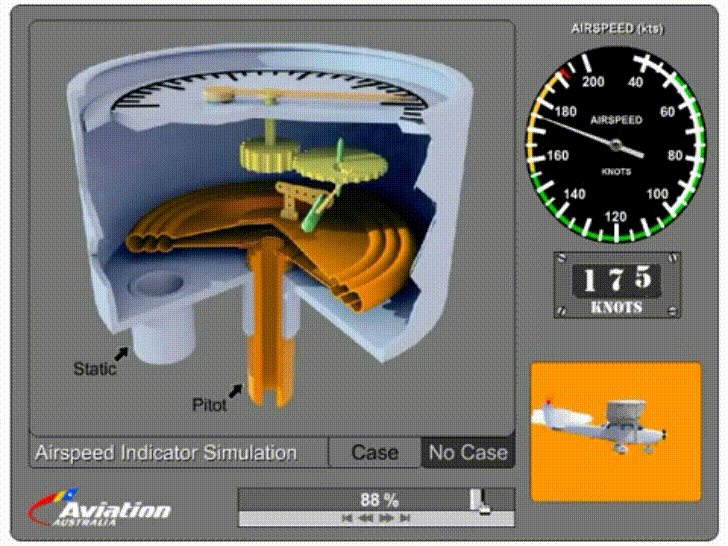
I understand the underlying principle of an airspeed indicator, ie. that it uses the Pitot-static system to measure the dynamic pressure as the difference between total pressure and static pressure.

Knowing that the dynamic pressure is $p_{d} = \frac{1}{2}\rho u^2,$ any linear variation in the measure will be a linear variation in $u^2$, not in $u$ alone. Yet the airspeed indicator markings are themselves uniformly spaced and still, they show $u$, not $u^2$. Therefore: how does the mechanical linkage convert a linear displacement (variation) in the dynamic pressure measurement $\propto u^2$ into $u$?

That is, how is the square root transformation of the dynamic pressure performed at the clockwork level of the airspeed indicator to obtain the indicated air speed?

My guess is that one of the gearwheels will be responsible for this but I haven't actually been able to find any information on this online.

Improve this question
$\endgroup$

2 Answers 2

Reset to default
4
$\begingroup$

enter image description here
(YouTube)

As the diaphragm inflates, it pushes a levered arm up, which drives a gear via a hollowed curved track (not via the cogs). The bigger the inflation, the smaller the rotation of the gear (arm increasing radius) compared to the piston displacement. It's the only variable in the mechanism that is not a fixed ratio.

Full disclosure: this is my analysis of the video, I haven't designed one before.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Do you reckon, in this example, that it is the shape of the hollowed track that does the square root transformation of the displacement then? $\endgroup$
    – VH-NZZ
    Commented Apr 2, 2018 at 7:46
  • 1
    $\begingroup$ @沖原ハーベスト - I do, it's the only variable in the mechanism that is not a fixed ratio. $\endgroup$
    – user14897
    Commented Apr 2, 2018 at 7:47
  • $\begingroup$ Ah. clever, indeed. Still, looks like a very fragile piece of machinery. I'd need to open an ASI one day. Thanks for the pointer to the video. $\endgroup$
    – VH-NZZ
    Commented Apr 2, 2018 at 7:50
1
$\begingroup$

Some early airspeed indicators were much simpler.

enter image description here enter image description here enter image description here

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .