How should we think of 'layers' of air flow?

Question

Do a search for "boundary layer" or "laminar flow" on this site, and you'll find plenty of things to read.

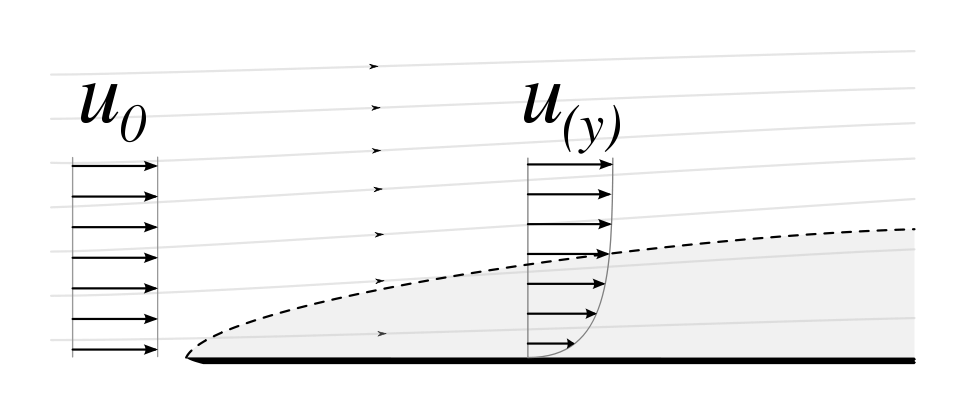
They'll talk about "the boundary layer", "flow separation" and so on, as though it were completely obvious what they actually were. Sometimes, they'll even be illustrated with diagrams representing the strata in the flow of air.

I'm no physicist, and this talk and these diagrams make me imagine the fluid equivalent of plywood, or expensive paper handkerchiefs, constructed of discrete layers of material that can be readily identified as such and peeled away from each other.

Is that really how these layers of moving air work? Is the boundary layer over an aircraft's wing like a discrete sheet, with a clear and distinct boundary between the next discrete layer? Or is there a smooth continuum, rather than a distinct step, between the layers and their properties?

Do distinct layers exist more in the conceptual analysis of aerodynamic behaviour than they do in readily identifiable physical entities?

If you were to plot a graph representing some property of the air flow (say its velocity, direction, pressure or turbulence) against distance from the surface of the wing, what sort of shape would the curve have?

In short: how should we imagine these layers?

Answer 1

To understand what a boundary layer is think about air moving above a flat plate. Before the plate and very far from it the air has everywhere the same speed and direction. However, directly on the plate its speed is zero: molecules directly on the plate do not move at all.

Between the plate surface where the air isn't moving and some distance from it where the air has a constant speed, there is a zone where the air changes its speed a lot. We call this zone boundary layer.

There isn't really any clear and distinct boundary delimiting it. That's why we have many definitions for its thickness. The dashed line in the image below is probably the 99% boundary layer thickness.

Generally we give a name to a layer when the physical effects and properties of the fluid in this concrete zone can be differentiated from the rest of the flow. Most of them are on fluid boundaries (body surfaces, places were fluids get gaseous,...) but you can have them inside a fluid too (shock waves).

For example, instead of looking at the velocity you could look at the temperature of a fluid flowing around a very hot body and define a temperature boundary layer.

Some known layers are:

• Laminar boundary layer
• Turbulent boundary layer
• Blasius boundary layer
• Stokes boundary layer
• Ekman layer
• Shock layer
• Knudsen layer
• Merged layer

Answer 2

For me the best comparison is with a multi-lane road. Imagine you drive along and all drivers stay in their lane. Then it will be possible that all the cars in one lane zip ahead while another lane crawls along.

Now imagine that drivers switch lanes frequently. The slow ones changing into a fast lane will force the others to brake, while the fast ones cutting into a slow lane will push the laggards forward.

Drivers who stay in their lane are like air molecules in a laminar flow. They follow a straight path and interact with their neighbors to the left and right mainly through shear, which will change their speed only slowly. On the other hand, air molecules in turbulent flow wiggle around all the time and bump into slower molecules, or get bumped by faster ones. This makes all of them assume the same speed, more or less. Only at the wall you find a strong speed gradient, maybe like at an onramp on a highway. The slow cars entering the highway speed up really fast when others will cut into their lane frequently.

Of course there are no lanes and no lane markings in air. Airflow is like a highway with an infinite number of infinitesimally small lanes, and instead of producing accidents, molecules which bump into each other are merely creating local pressure. The boundary layer which is mentioned so often is in the end a matter of definition: The boundary layer is defined to end when the local flow has reached 99% of the flow speed outside of the boundary layer. This allows to split the flow into a regime without viscous effects far from the body, which is easier to calculate, and one with viscous effects in a thin sheet around it. In reality there is no such thing, all air follows the same laws.

Answer 3

Think of the layers more as a liquid - imagine running water, just as there is sometimes a fast moving flow of water on the surface or in a reduced space, with slower moving currents beneath the surface.

Aerodynamics is actually an area of Fluid Dynamics, with air being a compressible fluid.

The Navier-Stokes equation can be derived to show mathmatically how the boundary layer actually works if you wish to invesigate further.