Suppose a plane has a cruise speed of 400 knots TAS. In a 4000 nm route we would have a round trip of 20 hours. If there was a jet stream of 100 knots in either direction, we would have 4000/500=8 hours in the tailwind direction and 4000/300=13.3 flying into the jet stream for a total of 21.3 hours. Now clearly, airlines are smarter than this and plan flightpaths, altitudes and speeds that maximize tailwinds and minimize time spent flying against the wind. The question, is to what extent does this strategy help? Does it simply offset the time lost compared to flying at the same speed in both directions? Or does it help even more than that. In other words, would a hypothetical windless world hurt the airline industry?
$\begingroup$
$\endgroup$
7
-
1$\begingroup$ Possible duplicate of Is head-on or trailing wind better? $\endgroup$– foootCommented Mar 10, 2016 at 17:48
-
2$\begingroup$ No, my question is whether winds help efficiency in the grand scheme of things/ $\endgroup$– user13363Commented Mar 10, 2016 at 17:58
-
1$\begingroup$ I believe it is clear that the second one $\endgroup$– user13363Commented Mar 10, 2016 at 18:46
-
4$\begingroup$ I'm puzzled by the number of votes to close, compared to frivolous questions that can be well received. IMO, this one, albeit a bit difficult to read, is really a good one, and it can be answered providing some insight. $\endgroup$– minsCommented Mar 10, 2016 at 19:46
-
1$\begingroup$ @mins Some of the close votes are for dupe close. However, I agree with you and voted leave open. $\endgroup$– reirabCommented Mar 10, 2016 at 19:49
|
Show 2 more comments
3 Answers
$\begingroup$
$\endgroup$
1
Yes, Jetstreams are a net benefit on longer flights because it is easy to minimize headwinds and maximize tailwinds for both directions.
Longer flights can detour hundreds of miles in order to take advantage of more favorable winds and still result in a net gain.
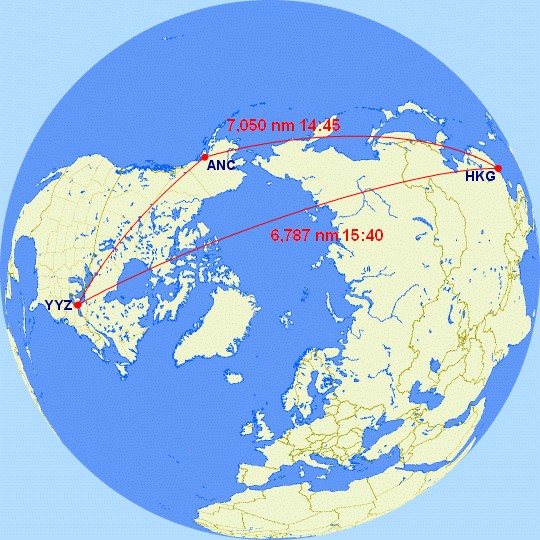
I frequently fly from Toronto to Hong Kong. We always fly a polar route YYZ-HKG as it is shorter, but usually take a HKG-YYZ North Pacific route over Alaska to take advantage of the prevailing winds.
This daily flight is always scheduled at 15 hours 40 mins for Toronto to Hong Kong over the pole but only 14 hours 45 mins returning via a longer route over Alaska.
The longer flight routing over Alaska is a net benefit in time and fuel due to the prevailing westerly winds over the North Pacific. This type of flexibility is not available on shorter flights.
YYZ-HKG 6,787nm non-Stop 15:40
HKG-ANC-YYZ 7,050nm non-Stop 14:45
answered Mar 10, 2016 at 11:55
-
$\begingroup$ As well as the benefit that the customers are usually happy with it. $\endgroup$ Commented Mar 10, 2016 at 13:32
$\begingroup$
$\endgroup$
3
The answer I'm about to give is purely theoretical, and many other factors are specific to Jetstreams, including the ability of a plane to navigate around them. However this is an interesting mathematical result indicating that in general winds are net negatives.
Let's imagine a plane flying a round trip 1000km each way at TAS 200kmh. In still air the trip takes 5hrs each way for a total of 10hrs.
For the same trip, with a 50kmh wind (headwind one way, tailwind the other), the tailwind leg takes 4hrs (1000/(200+50)). The headwind leg takes 6.66 hrs (1000/(200-50)). Total of 10.66hrs. The wind has a net negative effect. In our simplified world more time means more fuel, so there would be a negative on fuel consumption too.
The simple explanation is that the plane spends more time on the headwind leg, and less on the tailwind leg. The advantage on the tailwind does not cancel out the disadvantage on the headwind.
This answer has little bearing on actual reality, though it might apply to smaller aircraft without the ability to avoid winds. But it mainly illustrates a principle that may be useful in making rough time estimates of round trips.
-
4$\begingroup$ I realize how wind is a net negative if we do not do anything about it. My question was whether taking advantage of the wind factor by maximizing tailwinds and minimizing headwinds actually helps compared to no wonds a t all $\endgroup$– user13363Commented Mar 10, 2016 at 17:56
-
$\begingroup$ Even in small aircraft, we can and do adjust altitude and course to maximize benefit/minimize penalty for wind, just not as much as an airliner flying halfway around the world. $\endgroup$– reirabCommented Mar 10, 2016 at 19:47
-
1$\begingroup$ However in the case of the NATS, the wind is not the same for eastwards and westwards crossings, this provide an additional benefit. $\endgroup$– minsCommented Mar 10, 2016 at 19:50
$\begingroup$
$\endgroup$
11
The strategy helps in term of block fuel.
Case tailwind: at the same True Air Speed (TAS),you fly faster in respect to the ground, Ground Speed GS.
Case headwind: to keep the same TAS now your GS will be slower.
Let's compute the impact of Ground Speed / True Airspeed on Fuel Consumption, from Airbus getting to grips with Aircraft performance, page 131:
The specific range (SR) is the distance covered per fuel unit. Basically speaking, the specific range is equal to:
$SR_{ground} = \frac{GS}{FF}$
Considering Air Distance the specific range is equal to:
$SR_{air} = \frac{TAS}{FF}$
Where FF is the fuel flow [Kg/h], and TAS/GS is given in [NM/h].
So in a no-wind world, $TAS = GS$, otherwise at the same TAS constant, being the GS larger/smaller for a tail-/head- wind situation you will cover more/less distance with the same amount of fuel in respect to the ground.
In practice, operationally speaking, an aircraft tries to flies always at its most efficient speed, how speed effects the specific range can be seen in picture below (always from Aibus getting to grips with Aircraft Performance):
-
$\begingroup$ Drag depends on EAS, not TAS. There is EAS, $V_Y$, at which the drag is lowest and aircraft usually fly at that speed or only slightly faster. $\endgroup$ Commented Mar 10, 2016 at 10:05
-
$\begingroup$ Your assumption is wrong. Flights are not constrained by time. The schedule has enough buffer that the flight won't be late because of head wind and even if it was, nobody really cares. $\endgroup$ Commented Mar 10, 2016 at 10:08
-
$\begingroup$ And this does not answer the question anyway. At the very least a qualified estimate of how much the consumption is affected is needed. $\endgroup$ Commented Mar 10, 2016 at 10:09
-
$\begingroup$ @JanHudec Equivalent airspeed (EAS) is the airspeed at sea level in the International Standard Atmosphere at which the dynamic pressure is the same as the dynamic pressure at the true airspeed (TAS) here. The dynamic pressure at TAS and EAS are identical. Drag is proportional to Dynamic Pressure $D= q S Cd$. Q being the dynamic pressure, S the reference area and Cd the drag coefficient. So drag depends on EAS as much as TAS. $\endgroup$– GHBCommented Mar 10, 2016 at 10:20
-
$\begingroup$ @JanHudec an assumption, is just a simplification to show what factors play a role, in the last paragraph I tried to stress it out, probably not clearly enough, that in practice things are more complex, as you said, buffer etc. $\endgroup$– GHBCommented Mar 10, 2016 at 10:24