I believe I am beginning to understand how a leading edge vortex creates lift. Faster air creates a lower pressure above the wing making lift.
But I am hoping to get another view of this in terms of momentum.
Does this vortex draw in air from above, pulling it downward and as a result lifting the plane up? Or is the vortex shed downward off the tail of the wing? How does this work?
Does this vortex draw in air from above, pulling it downward and as a result lifting the plane up?
Yes, the bound vortex in combination with translational flow accelerates air downwards.
Or is the vortex shed downward off the tail of the wing? How does this work?
A vortex has no beginning and no end. When the wing begins to move, the vortex starts out of nowhere and has four parts: The bound vortex which travels with the wing, one parallel starting vortex which stays in place and two free vortices which connect both, running from the wingtips to the point where the wingtips were when the wing started moving. To be precise, an infinite number of vortices of infinitely small strength is created, and some cover only the center of the wing while others run over most of the wingspan.
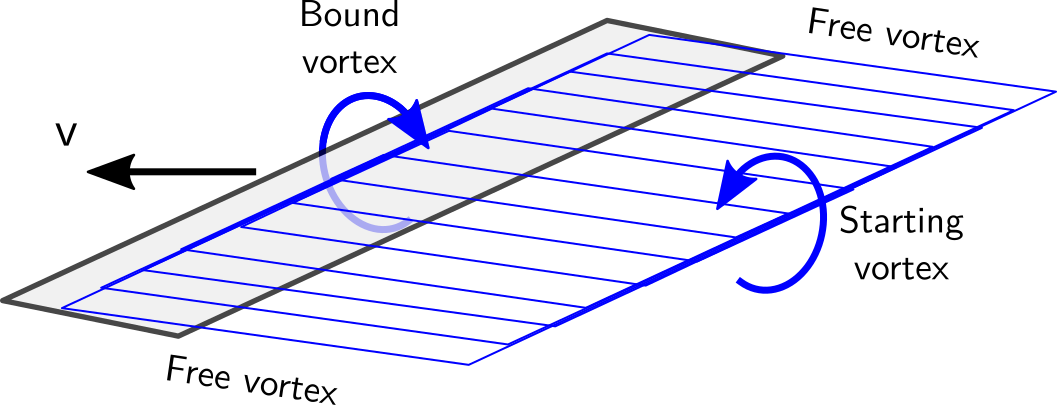
Idealized vortex structure (own work). The wing moves to the left with the speed v. The vortices are shown as discrete lines - to be precise, the bound vortices should be a sheet of infinitesimally small vortices which extend both over the chord and the span of the wing. Chordwise, they bunch together close to the leading edge, so their average streamwise position is at one quarter of the wing's chord.
And now let me give you my advice: If you do not want to operate or to write a potential flow code, do yourself a favor and forget all that. Read here why you must endure the vortex explanation of lift, and read here for a much more intuitive explanation of it all.
If by leading edge vortex you mean the swirling air coming off the leading edge of a highly swept wing, this is a result of flow separation. The inertia of the air molecules makes it impossible for them to flow around the leading edge, and they jump off the contour, leaving a volume of lower pressure behind. This pressure bends their flow path towards the wing and sucks the aircraft upwards. Since more air follows the same pattern downstream, the whole air above the wing starts to rotate while it flows downstream. This low pressure area sucks more air in from above, just like the regular low pressure region over a straight wing does.
Along and past the inner part of the wing this downward motion is prevalent and keeps the air spinning like the regular downwash from a straight wing.
