12
$\begingroup$

In another answer on SE Aviation it is mentioned that the ability to perform Pugachev's Cobra in a non-thrust-vectored aircraft needs "docile pitch behavior of the airframe up to approx. 110° angle of attack"

enter image description here

Can an F-16 perform Pugachev's Cobra?

What does "docile pitch behavior" mean in this context? Does it mean that when the control surfaces are returned to neutral the aircraft has a natural pitch down moment that returns it to its horizontal position?

If so, does the pilot actually have control over how long he can hold the vertical position in Pugachev's Cobra? What control surface enables him to exercise this authority in the post-stall regime when I'd have assumed the control surfaces lack such authority?

Or is the holding time of the vertical attitude in Pugachev's Cobra beyond the pilot's control and only related to some sort of "natural period" of this maneuver in that aircraft linked to how long it takes the restoring moment to return the aircraft to its nose down attitude?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
9
$\begingroup$

"Docile" means no big pitching moments over the angle of attack range. Since the control power with fully stalled control surfaces is rather small, the airframe must not create strong pitching moments, but keep a small pitch-down moment so the airplane returns eventually to normal angles of attack which allow regular flight.

The maneuver is dynamic, so the pilot needs to build up a specific pitch rate. Inertia will keep the aircraft pitching up, and the small pitch-down moment (and aerodynamic damping) will reduce the pitch rate so that the pitch-up stops when the nose is pointing straight up. What follows is the consequence of the small pitch-down moment: The aircraft slowly returns to controlled flight, and once control authority is re-established, the pilot stops the pitching motion.

By selecting the initial pitch rate, the pilot can control the maximum angle of attack, but once he leaves the flight regime of attached flow, he has to wait until inertia and aerodynamics do their part.

Improve this answer
$\endgroup$
12
  • 1
    $\begingroup$ @curious_cat: Yes. There might be more with specific elevator angles post-stall, but in essence that is it. $\endgroup$
    – Peter Kämpf
    Commented Jan 13, 2016 at 8:17
  • 1
    $\begingroup$ Also, how come there isn't a lot of altitude loss during the Cobra? Aren't we converting a streamlined, low drag attitude into essentially a large air brake + spoiler? $\endgroup$
    – curious_cat
    Commented Jan 13, 2016 at 8:27
  • 2
    $\begingroup$ @curious_cat Your high drag results in a speed loss primarily. $\endgroup$
    – Wirewrap
    Commented Jan 13, 2016 at 8:46
  • 2
    $\begingroup$ @curious_cat Don't forget the thrust vector. At the top of the maneuver you're getting esdentially no lift. The engines are maintaining altitude. And I think the loss of airspeed is the whole purpose of the maneuver (besides looking cool). Turns a pursuer into the pursuee $\endgroup$
    – TomMcW
    Commented Jan 13, 2016 at 22:19
  • 2
    $\begingroup$ @curious_cat: Elevator post-stall authority is very small. It will produce a drag force that is proportional to its projected area in streamwise direction. Say it is flying at 80° AoA. Rotating the fully-flying tail by -20° to 60° will change the projected area by (sin80°-sin60°)/sin80° = 12%. 12% less drag at the tail will add a small pitch-up increment. $\endgroup$
    – Peter Kämpf
    Commented Jan 13, 2016 at 22:32
0
$\begingroup$

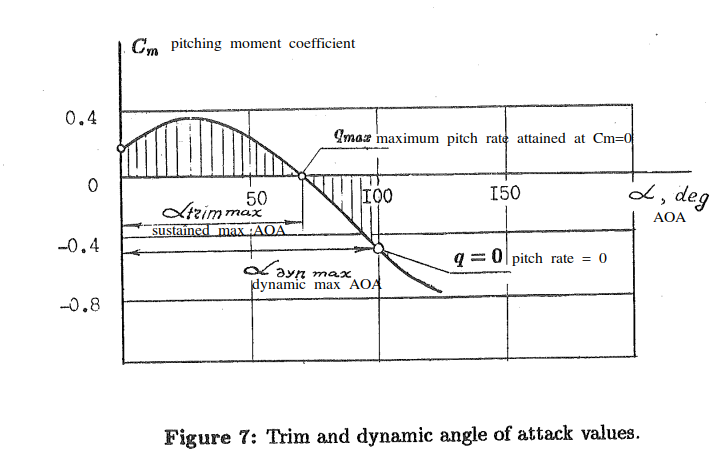
Continuing from this answer, the aircraft actually requires a strong pitching moment to maximize the shaded area of $C_m$ > 0 (nose-up moment) by full stabilator trailing edge up (TEU), as shown in the Su-27 Cm curve, in order to maximize pitch rate to get into the $C_m$ < 0 (nose-down moment) area as far as possible. The $C_m$ < 0 area above 70 deg AOA, where significant nose-down moment is mostly generated by the aircraft itself, allows pitch motion to be stopped and the aircraft to be recovered from the high AOA region naturally.

Su-27 Cm

Source: High Maneuverability Theory and Practice, TsAGI. (highly recommended)

So Why the area of $C_m$ > 0 matters? Because pitch acceleration is calculated as :

q-dot

Where the component of pitch acceleration due to aerodynamic moments related to $C_m$, as indicated in the equation is:

  • total $C_m$ * dynamic pressure * reference wing area * mean air chord / moment of inertia about the body Y axes $I_Y$.

Total $C_m$ also includes other moments, such as the pitch dampening moment $C_m$q, but I'll keep the complexity to a minimum extent.

Pitch rate (q) is simply the integration of q-dot. So a larger $C_m$ > 0 area would provide a greater pitch rate, if all the other variables are fixed. This would require the stabilator to be deflected and held to the max position as soon as possible, so the Direct Link control mode is used in the Su-27. Center of gravity is preferably at its aft limit to increase the area of $C_m$ > 0.

There's not much the stabilator can do once enough pitch rate is generated above the AOA limit, say, 24-26 deg AOA, where the maximum nose-down moment available (with full stab trailing edge down / TED) for stopping the pitch motion plummets, indicating a stalled stab.

The problem with the F-16 during a recovery is probably the deep stall around 60 deg AOA, as the full stab TED is not able to generate even a negative $C_m$, but rather, the aircraft would pitch-up. Pitch rate would converge and AOA can be stabilized at the deep stall trim point where $C_m$=0, in the positive static margin area of AOA > 50:

F-16 Cm

Source: NASA TP-1538. (also highly recommended)

But this would usually happen with a relatively low initial pitch rate. When recovering from a cobra maneuver, the negative pitch rate reaches its maximum at 60 deg AOA ($C_m$=0) with either a neutral or TED stab, and can easily overcome the pitch-up region. After AOA is reduced to 40 deg, where both static margin and $C_m$ are close to zero with a neutral stab, a pull on the stick may be required to stop the pitch down motion completely.

This all assumes that the FLCS is non-existent and we have direct control of the stabilator.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .