Determining Required Fuel from Payload/Range Diagram

Question

I would like to determine the amount of fuel required for a flight at a given range/payload combination - ideally from the payload/range-diagram [1] of the corresponding aircraft alone.

I know that for the case of maximum payload, the fuel required for a flight at a given range can be read off the extended payload-range diagram as (cf. [2], Section 3.3):

$$m_{fuel}=m_1-m_2$$

where $m_2=MZFW$ and $m_1$ can be computed as $m_2=((m_1-m_2)/R(A))*R$

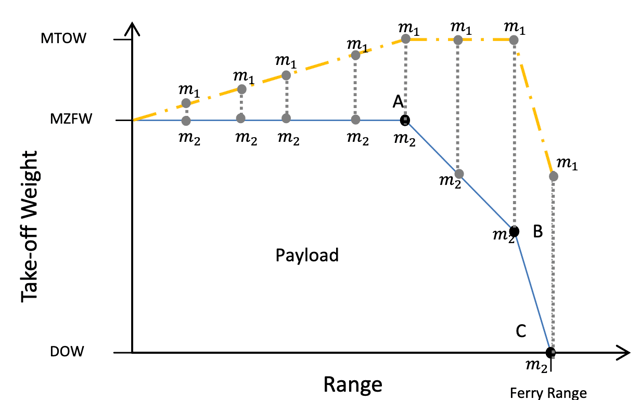
Image source: Figure 3.4 in [1]

But how can I read the fuel for an arbitrary payload that is smaller than the maximum payload (which is described in the diagram by the line up to point $A$)?

[1] Aircraft Fuel Consumption – Estimation and Visualization
[2] Aircraft Payload‐Range Analysis for Financiers

Answer 1

The gradient between points A and B of your diagram is determined by the overall efficiency of the airplane. It depends on the amount of fuel needed to fly a bit further by trading payload for fuel mass. Therefore, you will need to draw parallels to that line to the left and below it as shown in this diagram for a Boeing 777-200:

Boeing 777 range-payload diagram (picture source)

Note that the distance between lines for the same step reduction in take-off weight increases as you move leftward because the remaining fuel necessary to finish the flight needs to be carried over shorter distances.

To find the fuel mass for an arbitrary payload and a given range you need to draw a horizontal line at the right height of the OEW plus payload scale and then move rightward until you are above the desired range on the X-axis. The brake release gross weight for this payload-range combination can then be interpolated from the diagonal lines.

Of course, you can as well use the different corner points of the diagram and find the right values of the Breguet range equation and modfify those to arrive at the solution.