What is the definition of "geometric height" referred in the ISA Model?

Question

The International Standart Atmosphere document always mentions the "geometric height" (along with the geopotential hieght) in their calculations. I predict that it means orthometric height, which is the distance from a point in the atmosphere to the Geoid (as an approximation of the Mean Sea Level). My questions are:

1. Is it actually the same as orthometric height? Is there a reliable source with the definition? I need to be certain because I am going to be using these formulas in a critical setting. There is no definition I could find in the document and other sources that I found have been unreliable.
2. If they are the same, why don't they use the term "altitude" instead of "height" since they're referring to the distance to Mean Sea Level and not to the Earth's surface?
3. If they are not the same, how are they different? And again, is there a reliable source that has the definition.

Thanks in advance.

Answer 1

In the ICAO Standard Atmosphere model, as described in ICAO Doc. 7488, the geometric height is equivalent to the orthometric height.

The ISA model uses a hydrostatic equation to calculate the pressure differential over a geopotential height difference.

The reference height (0 meters) of the model is the mean sea level. It furthermore assumes a standard acceleration due to gravity, conforming to a latitude of 45.32'33". This $g_0 = 9.80665$ m/s² is used to convert between geopotential height $H$ to geometric height $h$.

For this purpose the ISA model assumes a non-rotating earth with uniform mass distribution, so that only Newton's gravitation law can be used to obtain the relation between $H$ and $h$:

$H=\frac{rh}{r+h}$.

with $r = 6356766m$ the radius of the nominal (spherical) earth.

Now to orthometric height.

The orthometric height of a point is the distance H along a plumb line from the point to a reference height (typically the mean sea level).

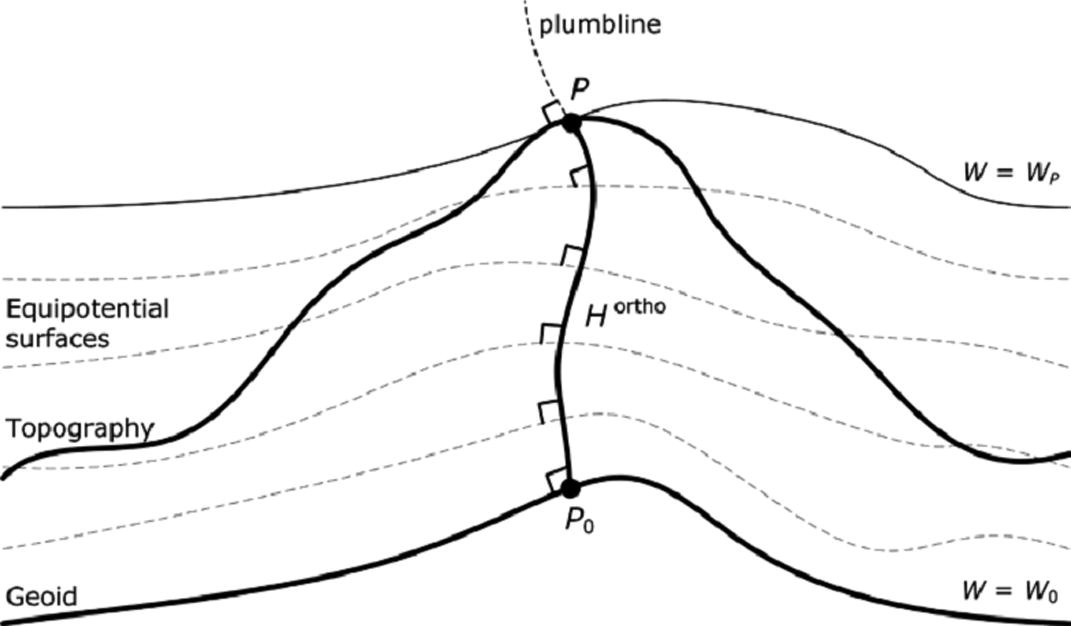
_{Image source: ScienceDirect: Evaluation of the various orthometric height systems and the Nigerian scenario – A case study of Lagos State}

The plumb line however, is not a straight line. Due to rotation of the earth and gravitational anomalies due to an irregular mass distribution in the earth, the plumb line curves.

Within the ISA model, orthometric height and geometric height are the same, because the model assumes the earth is uniform, spherical and non-rotating. Therefore the plumb line in the ISA model is straight.

In the real world however, the orthometric height (measured along the curve of the plumb line) is thus not equal to the geometric height (which measures along a straight line).

The difference are extremely small; for practical purposes this can be ignored in most fields and orthometric height is usually said to be the same as geometric height.

Answer 2

1. Geometric height differs from your provided definition of orthometric height.

geometric altitude is the standard direct vertical distance above mean sea level

is the definition cited from your 1976 source by https://en.wikipedia.org/wiki/International_Standard_Atmosphere.

2. Word usage can drift over the decades (height vs. altitude). This may be a separate, more general, question. Section 1.2.6 and Table III of your source calls it Geometric Altitude, though.

3. They differ in that your source nowhere mentions the geoid, so it can't be using your definition of orthometric height.