Why does the aspect ratio of a wing become less important at supersonic speeds?

Question

My understanding is that an increase in the aspect ratio (AR) of a supersonic wing won't increase the efficiency like it would for a subsonic wing.

If the subsonic induced drag is: $C_{D_i} = (C_L^2)/(\pi\times AR\times \epsilon)$

And the supersonic lift wave drag for slender bodies* is: $C_{DWL} = 2\times \frac{C_L^2}{\pi\times AR}$

They're both proportional to $\frac{1}{AR}$ so why is the aspect ratio considered insignificant at supersonic speeds? ------> It seems like I'm missing something here.

*Does this term only apply things like fuselages and nacelles and not wings? If so, what does it mean for it to have an aspect ratio?

Peter Kampf please help

Answer 1

The two main reasons for wings with a subsonic leading edge are:

1. The lower lift coefficient in supersonic flight, and
2. the need to keep wing thickness down in order to minimize wave drag in supersonic flight.

Your observation is correct: The lift-related drag coefficient is proportional to the inverse of the aspect ratio, regardless of flight regime for slender bodies. In all cases, lift is created by accelerating air downwards, and efficiency is better when more air can be accelerated by less.

But another factor is the lift coefficient, the square of it to be precise. Again this influence on drag is equal for sub- and supersonic flight, but the magnitude of the lift coefficient isn't. Take the XB-70, for example (source):

• Cruise lift coefficient: 0.1 to 0.13, $c_L^2$ = 0.01 to 0.0169
• Takeoff lift coefficient: 1.3 to 0.73, $c_L^2$ = 1.69 to 0.533
• Landing lift coefficient: 0.626, $c_L^2$ = 0.392

Even at the highest supersonic cruise lift coefficient, the approximated lift-dependent drag coefficient is only 4.3% of what it is at landing speed. Supersonic drag is dominated by pressure and friction, and the cost of lift creation is dwarfed by the other sources of drag. Or let's look at another aircraft designed for sustained supersonic flight, or more specific, it's flight envelope (source):

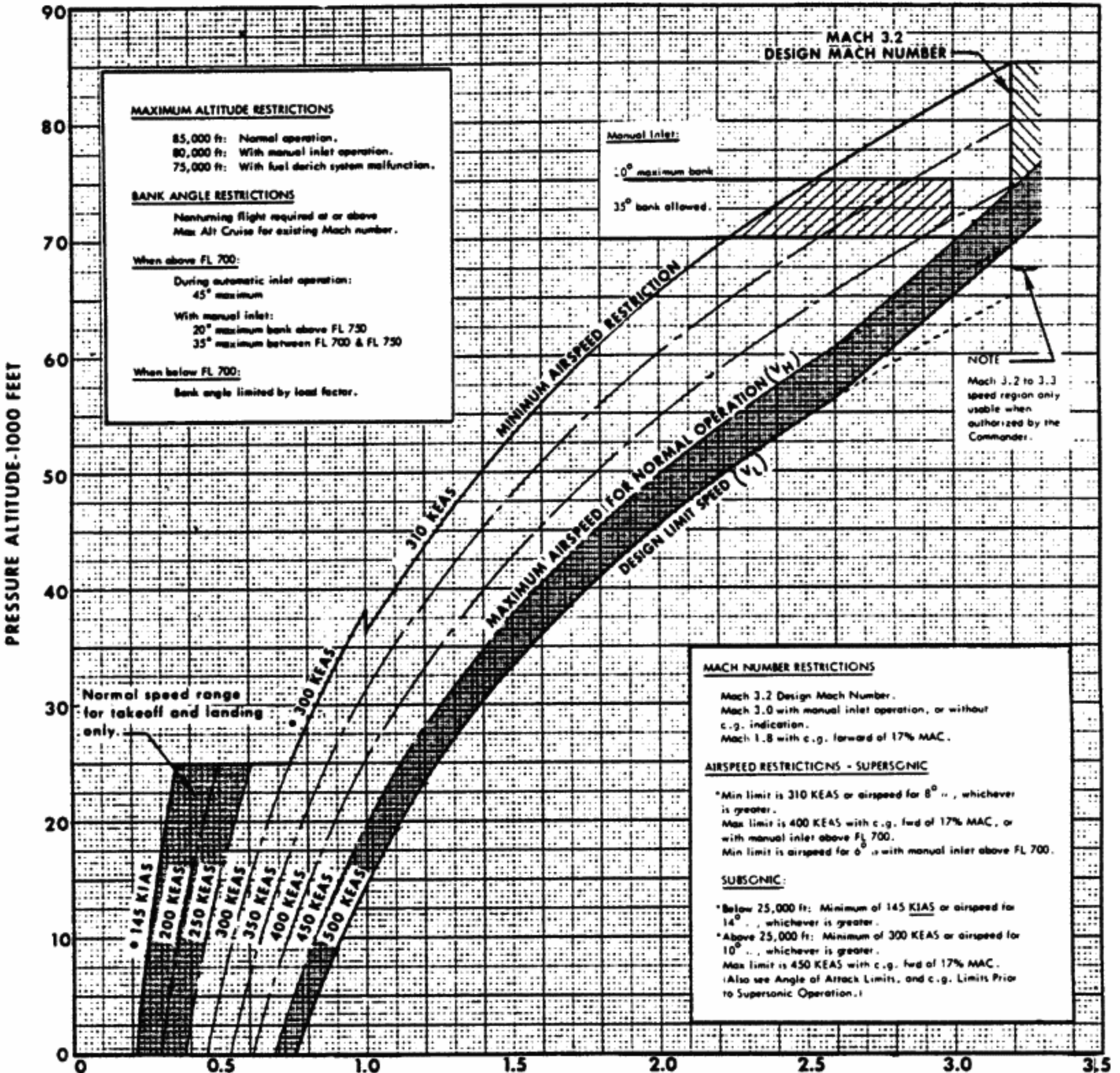
The aircraft operates in a narrow band of dynamic pressures, trading density for speed to stay within a corridor of 310 KIAS to 450 KIAS when flying at supersonic speed. Here the lift coefficient is roughly between 0.1 and 0.3 (depending on aircraft mass and load factor) and again much lower than at low speed, where minimum speed drops to 145 KIAS.

Note that the lift wave drag formula for slender bodies is only strictly true for $AR$ approaching zero. At higher aspect ratios and especially with a supersonic leading edge this relationship is diminished. In supersonic flow the aspect ratio only comes into play by influencing the part of the wing that is within the Mach cone of the wingtip. In other words: The supersonic flow around a slender body is everywhere influenced by tip effects, whereas the inner and forward part of a higher aspect ratio wing experiences two-dimensional flow, being blissfully unaware of lateral limitations to wingspan.

Now to the structural factor: Wave drag has two components, one from lift and the other from the displacement effect. Pushing air out of the way at supersonic speed must be avoided as much as possible, because it is doubly punished: Surfaces facing in flight direction experience higher pressure than ambient, and those facing backwards experience lower pressure (i.e. suction). In all, zero-lift wave drag increases with the square of the thickness ratio, so there is no space in supersonic wings for the beefy spar a high aspect ratio wing would require.