TLDR: Running the numbers with some assumptions, an average person in average conditions cannot induce a parachute stall by jumping out of the basket
I'm no ballooning expert, but I can give some reasoning. This relies heavily on the AAIB report found here
Background information on how balloons can crash
Normally, balloons have a parachute at the top of the balloon. Normal balloons are designed such that once you open the parachute, the flow of air that goes along it, generates lift, which pushes up the parachute and closes off the gap. This means it's a self-restoring element. However, this only works if the parachute can 'use' the airflow to generate enough lift to push itself back up. Like a normal wing, the parachute can end up in a condition where the flow stalls and lift is greatly reduced, meaning, the parachute cannot return to its closed position.
This is called parachute stall . Similar to wings, a stall is dependent on the exact flow conditions and the geometry, so I cannot say in a generic way when it will happen.

Source
Analysis on what can factors influence parachute stall
However, we can look at what's influencing the forces on the parachute.
Let's look at a parachute in normal conditions. It looks like this:
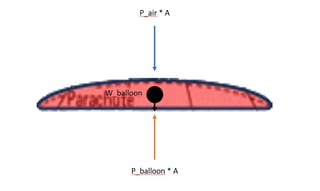
If we look at the balance of the forces in z on the parachute:
$$ p_{air} \cdot A + W_{balloon} = p_{balloon} \cdot A \tag{1}\label{1}$$
or reorganized:
$$ W_{balloon} = \left(p_{balloon} - p_{air}\right) \cdot A \tag{2}\label{2}$$
If we want to let some air out, we can pull the rope, which will reduce $A$, and reduce the net upward forces due to the pressure difference, causing the parachute to fall.
However, the force balance of Formula \eqref{1} only holds when we're not moving vertically. If we go up at a certain speed, the top of the parachute will collide with the air, causing an increase in pressure, called dynamic pressure:

The dynamic pressure is added to the force balance of \eqref{1}:
$$ p_{air} \cdot A + W_{balloon} + p_{dyn} \cdot {A} = p_{balloon} \cdot A \tag{3}\label{3}$$
The dynamic pressure is related to the flow velocity V:
$$ p_{air} \cdot A + W_{balloon} + \frac{1}{2}\rho V^2 \cdot {A} = p_{balloon} \cdot A \tag{4}\label{4}$$
So, rising (by the pilot, or by a passenger jumping out), can cause the parachute to fall, and then it's dependent on the exact conditions if a stall occurs or not. If a stall occurs, the parachute will not return to its closed position, and the balloon will let out hot air and crash.
This is confirmed by AAIB:
High rates of climb: With an increase in the rate of climb comes a corresponding
increase in the pressure on top of the balloon envelope and above the parachute.
The faster the climb rate, the higher the pressure. As this pressure increases,
the difference between the pressure above and below the parachute valve
decreases, resulting in a decrease in the distance the parachute can be pulled
into the envelope before it reaches the stall point. Aggressive venting whilst in
a fast climb could more easily result in a parachute stall.
Of course, with the pilot unaware, you could have a combination of the two, where the pilot is making the balloon rise quickly and then somebody jumps out, which would exacerbate the risks.
Given Formula \eqref{4},the right input data and an estimation of how much $\Delta V$ one person jumping would generate one could estimate the risk of parachute stall.
However, without knowing more about the exact flow conditions and geometry of the parachute, we cannot determine if it's stalled.
Estimating risk due to person jumping
What we can do, is to look at the vertical speeds that have led to parachute stall, and then see if a person jumping out could induce those velocities.
From the document we find the vertical velocity where a parachute stall happened:
The AAIB obtained flight data from one case involving a lightly loaded balloon that climbed
from a low height at 8 m/s, a similar rate to G-CMFS’s last climb before the accident. The
pilot described a parachute stall which caused the throat to close. The balloon stopped
climbing and began descending at about 6 m/s (1,200 ft/min). He managed to burn through
the envelope to re-inflate it and landed shortly afterwards.
Can a person jumping out of the balloon lead to such a vertical velocity?
This source gives the following mass for a hot air balloon with 5 passengers: 800 kg, with a passenger weight of 80 kg each.
Once the passenger jumps, there is an upward imbalance of 80 * 9.81 = 784.8 N. But what is the 'terminal velocity' achievable by this imbalance?
We can set the upward force equal to the drag.
$$ F_{up} = \frac{1}{2} \cdot \rho \cdot V^2 \cdot C_D \cdot A $$
$C_D \cdot A$ is 254m2 for a typical hot air balloon in ascending flight.
At a typical flight altitude of 3,000 ft, the density is 1.12 kg/m3
$$ 784.8 = \frac{1}{2} \cdot 1.12 \cdot V^2 \cdot 254 $$
Solving for V gives us an upward terminal velocity of 2.34 m/s; less than the mentioned 8 m/s needed for parachute stall.
So the average passenger of the average hot air balloon in average conditions, with a non-responding balloon pilot, can not make it crash by jumping out.



