How does the engine RPM vary in this process?
It is safe to say that in most operating conditions the shaft-speed will increase with higher thrust settings. Here is why:
When we look at a very simplified version of the processes in a jet-engine, increasing fuel-flow will increase temperature in the combustor. This will push point 4 to a higher T.
[![https://www.grc.nasa.gov/www/K-12/airplane/brayton.html][1]][1]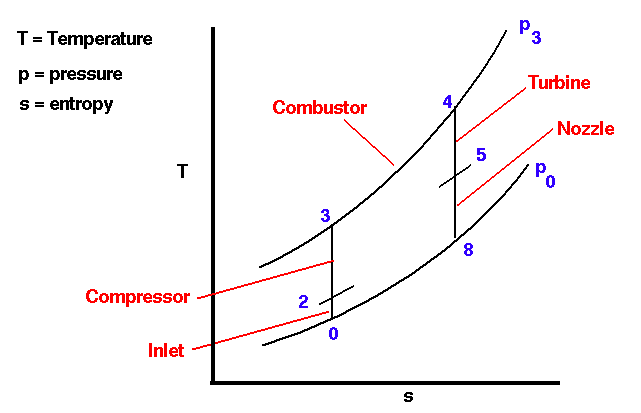
At first the turbine will consume the same amount of energy. This means the »distance« between point 4 and 5 will stay the same. Finally, this means the »distance« between point 5 and point 8 is bigger. In a T-S-diagram the distance is a measure of energy stored in the air, so there is more energy in the air exiting the nozzle so the jet will be faster and this will increase the airspeed. BUT, another thing is happening as well, actually it is happening simultaneously but a bit slower: since the air is hotter at the turbine entry the turbine will also extract more energy from the hot air and will therefore speed up. Which leads to a higher speed in the compressor as well, which leads to your second question.
Is there an equation which binds together spool RPM to the thrust?
The short answer is: No
But, actually it is not that easy. There exits no single equation for the engine but a system of equations. The character of this system is non-linear an holds a large number of parameters (e.g. compressor- and turbine-efficiency curves) which are sometimes unknown and need to be measured during an experiment.
However, it is possible to perform experiments and reduce the number of parameters and build correlations. Which is done regularly in order to model engine performance or control the engine.
I am not entirely sure what kind of »equations« you were referring to in your question. Because in principle correlations are equations as well but one needs to fit those correlations based on experiments to determine the parameters which you cannot know in advance.
The above is a very simplified view on a jet-engine. Depending on the type on jet-engine there are a lot more settings which influence the engine-behaviour: for example the Variable-Guide-Vane-Actuation-Law, or the Bleed-Air-Schedule, or the Cooling-Sytem of the casing. They all contribute to the complexity which make a »simple equation« impossible. [1]: https://i.sstatic.net/9UMKY.png
